Fretboard Harmony 3
By Peter Altmeier-Mort
For those readers who made an effort to analyze example 1 from the last Fretboard Harmony column, the answers are:
Share this:
[feather_share]
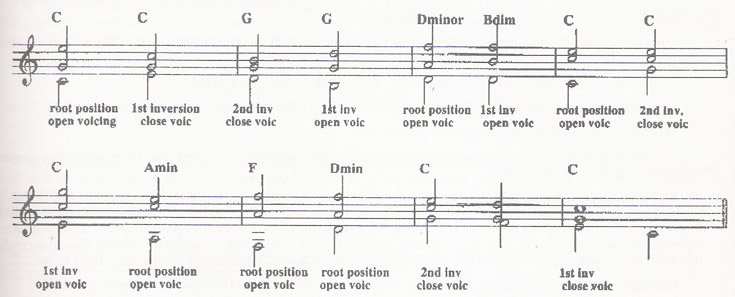
The second last chord, which has been left unnamed here, was one of several misprints in the last installment.This chord should have been F, B, D, making it an inverted diminished triad. The indicated chord of F, G, D, will in fact be covered in the material set out below. See if you can identify it at the conclusion of this issue’s lesson.
The Movement of Voices
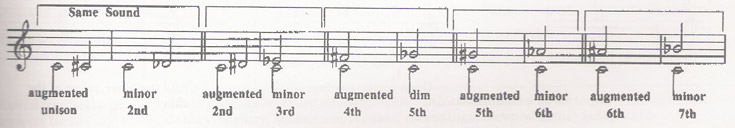
As stated in the first article of this series, it is the way in which intervals “resolve” or move from one pair to another that affects the way chords and some of their individual voices move one to another. This is one reason why the subject of intervals is treated before chords when studying theory of music, yet it never seems to be made abundantly clear to students, especially those who may be preparing for theory exams. Beginners, and intermediate students, should get to know the following tables of intervals (done in the key of C major). The bracketed numbers underneath show the distance between each note of the interval in tones.
- Unison = the combined sound of two notes of the same pitch.
- Perfect = a term (based on mathematical ratios) applied to intervals of the octave, 5th and 4th.
- Octave = the interval between the 1st and 8th notes of a scale, or any two notes of the same letter name 6 tones apart (from the Latin “octo” = 8).
Taking this one step further, the above basic major intervals can be altered with the use of sharp and flat signs to produce finer gradations of intervals within this range.
- Augmented = enlarged, made bigger – used on those major or perfect intervals that can be made larger by one semitone (sharpening the upper note).
- Diminished = reduced, made smaller – used most commonly on the perfect 5th and 4th intervals to make them smaller by one semitone (flattening the upper note).
As will be seen in the near future, it is from this spectrum of intervals we form the more complex chords like 6th and 7th chords (9th and 13th chords too) augmented, diminished and flattened 5th chords etc.
DISSONANCE in music means “unstable.” A dissonant interval (or chord) has a tendency to ‘resolve’ or move towards something more stable. This characteristic in harmony is often termed TENSION.
CONSONANCE in music can be defined as “stability.” Consonant intervals are pairs of notes which sound compatible or ‘agreeable’ together. This is termed FUSION.
The foundation of harmonic music is a continual interplay between fusion and tension, or consonance and dissonance. The categories of fusion and tension concerning intervals are:
1) Consonances – unisons, octaves, 5ths, 4ths, major and minor 3rds and 6ths.
2) Neutral – augmented 4ths or diminished 5ths.
3) Mild dissonances – minor 7ths and major 2nds.
4) Strong dissonances – major 7ths and minor 2nds.
The student should select the appropriate intervals from the above tables and play through these categories of fusion and tension on the guitar.
The first chord with an inherent tension or dissonance that students experience in their music is that of the Dominant 7th. This is the triad built on the 5th note of a scale with an interval of a 7th (above this root) added to it. In the key of C major the 5th (or dominant) note of the scale is a G. Counting on up through the scale from here, the 7th note above this G is an F. This is an interval of 5 tones and it is in fact a minor 7th – a mild dissonance. And so it is with all chords called ‘a 7th chord,’ the basic major triad has an interval of a minor 7th added to it.
Play this interval on the guitar, listen to it, and then add the notes of the basic triad built upon G.
This is the chord of G7, the dominant 7th chord of the key of C major. When writing the notes of this chord, the 5th note of the triad (a D here) is often omitted to make way for a 3 or 4 part writing of the chord with the extra note of the added 7th.
Because of the “tension” this minor 7th interval creates in the dominant 7th chord, it has to resolve or move to a more stable interval (or chord) after it has been heard (at least in traditional harmony this is the case). In the following chord progression the G7 chord moves onto the C chord. The upper note of the interval of the 7th in the first chord falls a semitone and this is the most common resolution for the dissonance or tension created by the 7th chord.
The G7 chord could also resolve to another chord from the key of C, an A minor chord for example. Again the dissonance of the 7th interval can be resolved by falling a semitone.
If G7 was to move to another chord from the key of C which itself contained an F note as the G7 chord does, no resolution by a semitone drop would be necessary. Notes which are common to any two connecting chords do not move, and this is known as a passive resolution of the dominant 7th chord.
For example:
With the following intervals and resolutions, fill in the missing voices of the chords involved and label each chord with its correct name. Remember to leave out the 5th of the dominant 7th’s basic triad. Also, the resolving 7th will not always appear as the upper most note of the chord. After writing in your answers, play them on the guitar to hear how they sound.